|
|
Developmental Biology - Morphogenesis
The Beautiful Simplicity of Tissue Formation
There are rules that govern embryo development, guiding the branching of tissues into organs...
In the 100th year of publication of a seminal treatise on the physical and mathematical principles underpinning nature - On Growth and Form by D'Arcy Wentworth Thompson - a Cambridge physicist has led a study describing an elegantly simple solution to a puzzle that has taxed biologists for centuries: How complex branching patterns of tissues arise.
Branching patterns occur throughout nature - in trees, ferns and coral, for example - but also at a much finer scale, where they are essential to ensuring that organisms can exchange gases and fluids with the environment efficiently by maximising the surface area available.
For example, in the small intestine, epithelial tissue is arranged in an array of finger-like protrusions. In other organs, such as kidney, lung, mammary glands, pancreas and prostate, exchange surfaces are packed efficiently around intricate branched epithelial structures.
"On the surface, the question of how these structures grow - structures that may contain as many as 30 or 40 generations of branching - seems incredibly complex," explains professor Ben Simons PhD, University of Cambridge, Cavendish Laboratory and Wellcome Trust/Cancer Research UK Gurdon Institute — and leader of the study published in the journal Cell.
The classic problem of 'branching morphogenesis' has attracted the attention of scientists and mathematicians for centuries. Indeed, the mathematical underpinnings of morphogenesis — the biological process causing organisms to develop their shape — was the subject of Wentworth Thompson's classic text published in 1917 by Cambridge University Press. Thompson had been a student at Cambridge studying zoology at Trinity College, and briefly worked as a Junior Demonstrator in Physiology.
During development, branching structures are orchestrated by stem-like cells that drive ductal growth and division (or 'bifurcation'). Each subsequent branch will then either stop growing, or continue to branch again.
In a study published in Nature earlier this year, Simons, in collaboration with Jacco van Rheenen, Hubrecht Institute in Utrecht, the Netherlands, showed that in the mammary gland, these processes of division and termination occur randomly — but with almost equal probability.
"While there's a collective decision-making process going on involving multiple different stem cell types, our discovery that growth occurs almost at the flip of a coin suggested that there may be a very simple rule underpinning it."
Benjamin D. Simons PhD, Cavendish Laboratory, Department of Physics, The Wellcome Trust/Cancer Research UK Gurdon Institute and The Wellcome Trust/Medical Research Council Stem Cell Institute, University of Cambridge, UK.
Professor Simons and his colleague Edouard Hannezo PhD, observed that there was very little crossover of the branches - ducts seemed to expand to fill the space, but not overlap. This led them to conjecture that as ducts grow and divide — as soon as a tip touched another branch, it would stop.
"In this way, you generate a perfectly space-filling network, with precisely the observed statistical organization, via the simplest local instruction: you branch and you stop when you meet a maturing duct. This has enormous implications for the basic biology. It tells you that complex branched epithelial structures develop as a self-organized process — reliant upon a strikingly simple, but generic, rule, without recourse to a rigid, pre-determined sequence of genetically programmed events."
Edouard Hannezo PhD, Cavendish Laboratory, Department of Physics; The Wellcome Trust/Cancer Research UK Gurdon Institute; The Wellcome Trust/Medical Research Council Stem Cell Institute, University of Cambridge, UK, and a Sir Henry Wellcome Postdoctoral Fellow based at the Gurdon Institute.
Although these observations were based on the mammary gland epithelium, by using primary data from Dr Rosemary Sampogna at Columbia University, Professor Anna Philpott in Cambridge and Dr Rakesh Heer at Newcastle University, the researchers were able to show that the same rules governed the embryonic development of the mouse kidney, pancreas and human prostate.
"In the mammary gland, you have a hundred or more fate-restricted stem-like cells participating in this bifurcation-growth-bifurcation process, whereas in the pancreas it's just a handful; but the basic dynamics are the same," says Professor Simons. "The model is aesthetically beautiful, because the rules are so simple and yet they are able to predict the complex branching patterns of these structures."
The researchers say their discovery may offer insights into the development of breast and pancreatic cancer, where the earliest stages of the disease often show an irregular tangled ductal-like organization.
"A century after the publication of On Growth and Form, it's exciting to see how the concepts of self-organization and emergence continue to offer fresh perspectives on the development of biological systems, framing new questions about the regulatory mechanisms operating at the cellular and molecular scale," adds Professor Simons.
While it may be too early to tell whether similar rules apply to other branched tissues and organisms, there are interesting parallels: branching in trees appears to follow a similar pattern, for example, with side branches growing and bifurcating until they are shaded or until they are screened by another branch, at which point they stop.
"This is an elegant study that helps us to understand what guides the decisions our cells make during essential developmental processes. It's fascinating to see that such simple rules can govern the generation of such highly complex patterns and that these rules can apply to different branched structures."
Chiann-mun (Sheny) Chen PhD, Wellcome Trust, Cellular and Developmental Science.
Highlights
• Branching morphogenesis follows conserved statistical rules in multiple organs
• Branching morphogenesis follows conserved statistical
• Ductal tips grow and branch as default state and stop dividing in high-density regions
• Branching morphogenesis follows conserved statistical
• Model reproduces quantitatively organ properties in a parameter-free manner
• Branching morphogenesis follows conserved statistical
• Shows that complex organ formation proceeds in a stochastic, self-organized manner
Summary
The morphogenesis of branched organs remains a subject of abiding interest. Although much is known about the underlying signaling pathways, it remains unclear how macroscopic features of branched organs, including their size, network topology, and spatial patterning, are encoded. Here, we show that, in mouse mammary gland, kidney, and human prostate, these features can be explained quantitatively within a single unifying framework of branching and annihilating random walks. Based on quantitative analyses of large-scale organ reconstructions and proliferation kinetics measurements, we propose that morphogenesis follows from the proliferative activity of equipotent tips that stochastically branch and randomly explore their environment but compete neutrally for space, becoming proliferatively inactive when in proximity with neighboring ducts. These results show that complex branched epithelial structures develop as a self-organized process, reliant upon a strikingly simple but generic rule, without recourse to a rigid and deterministic sequence of genetically programmed events.
Authors
Edouard Hannezo, Colinda L.G.J. Scheele, Mohammad Moad, Nicholas Drogo, Rakesh Heer, Rosemary V. Sampogna, Jacco van Rheenen and Benjamin D. Simons
Acknowledgements
The research was funded by the Wellcome Trust with additional core support from Cancer Research UK and the Medical Research Council.
Return to top of page.
| |
|
Sep 17 2019 Fetal Timeline Maternal Timeline News
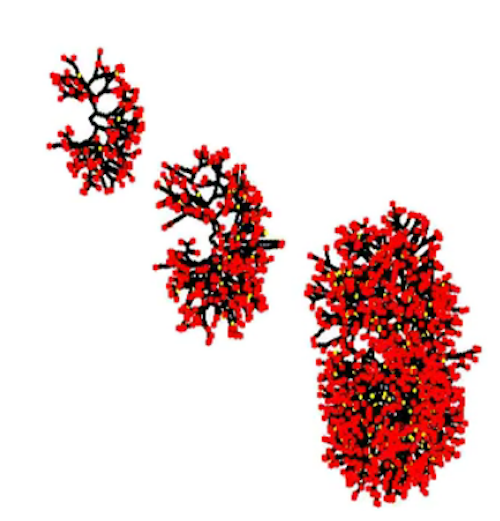 Branching process of the kidney. Red tips are still actively proliferating. In the kidney, ductal termination is accompanied by the appearance of nephrons (the functional unit of the kidney), in yellow. CREDIT Edouard Hannezo, University of Cambridge.
|



